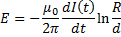1. A conducting bar of resistance R slides frictionlessly with a constant speed upward over  a pair of resistanceless rails inclined at an angle 2q under the action of some external force. A uniform magnetic field of strength B subsists in the region perpendicular to the plane of the rails as shown. Find the current induced in the circuit both by direct use of Faraday’s law and by explicit use of Lorentz force for calculation of motional emf.

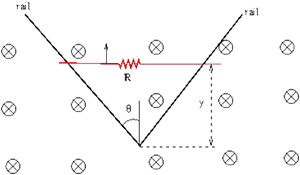
From geometry, we have, 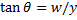 where 2w is the instantaneous length of the bar enclosed by the circuit and y the distance  from the junction of the rails. The area swept by the bar is

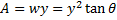
The emf, as given by Faraday’s law is

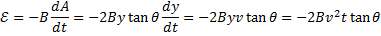
assuming that the bar started sliding at t=0 from the junction. The current is obtained by dividing this expression by R.

Since only the bar is moving, the motional emf is only along the sliding bar and is given by 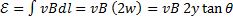, which gives the same result as above.

2. A rectangular conducting loop of width w , length L  and resistance R falls under gravity keeping its plane always vertical . An inhomogeneous magnetic field 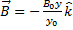 points into the page (xy) plane).  Find the terminal velocity of the loop.

At a time t, when the centre of the loop is at the position y, the flux threading the loop is given by .  The emf is given by . The current direction is counterclockwise as per Lenz’s law. Let us look at the problem from Lorentz force point of view. The force on the current is . The force on the two sides cancel, being equal and opposite.  The vertical forces are . The terminal velocity occurs when the net force is zero, so that the current in the loop should be , which requires the terminal velocity to be

3. A circular loop  of radius R rotates with an angular speed 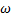 in a uniform magnetic field directed along the x axis. The loop rotates about an axis  parallel to the z axis.  Calculate the emf induced in the loop.

If 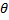 is the instantaneous angle between the normal to the loop and the direction of the magnetic field,

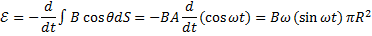
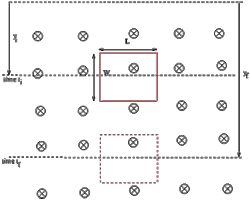
4. A long coaxial cable consists of a thin wire surrounded by a concentric shell of radius R. Current goes up through the wire and returns through the shell. If the current varies with time at a constant rate 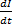, calculate the emf developed and the induced electric field  in the rectangular loop of side  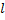shown in the figure.

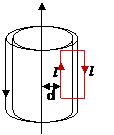
We assume that the instantaneous field is given by the corresponding steady state expression, I.e. the magnetic field at a distance r  for a long straight wire is given by 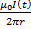. Thus the flux through a strip at a distance r from the wire  between the wire and the she shell is  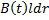so that the total flux is (since the field outside is zero),

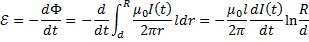
Since the electric field can only depend on the distance,

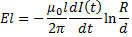
which gives,